portfetish.blogg.se - Convert lat long to xy

#Convert lat long to xy software
#Convert lat long to xy code
#Convert lat long to xy professional

Would a loop that iterates through each row be inefficient. The issue is if I have a large data frame or list ( >100,000 rows) of coordinates. This works fine if I want to convert between single points. Return (r * np.radians(lam) * cos_phi_0, r * np.radians(phi))

I'm not sure if a loop that iterates over each row is the most efficient way to do this? Below is the script that converts a single XY point. I want to do something similar on a larger df. However, I have to perform this for specific points at each point in time ( row by row).

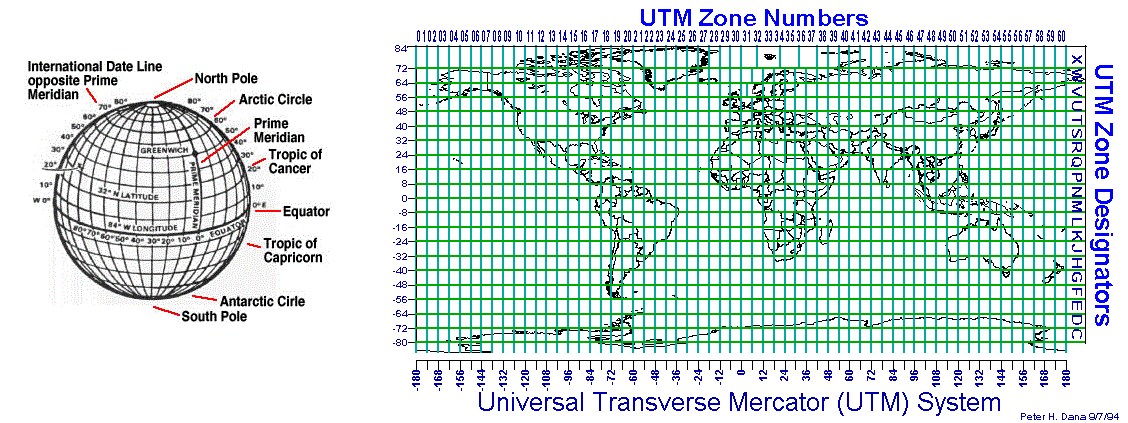
#Convert lat long to xy professional

Or if you have some level professional GPS has Altitude H ( msl,heigh above mean sea level) and UNDULATION,the relationship between the geoid and the ellipsoid (m) of the chosen datum output from internal table.I have a working script that converts Latitude and Longitude coordinates to Cartesian coordinates. This is done by interpolating a grid of the geoid height file with a spatial resolution of 15 arc-minutes. The MSL height has to be converted to height h above the WGS 84 ellipsoid by using the geopotential model EGM96 ( Lemoine et al, 1998). Usually GPS will give us H of above MSL height.

sttransform does this for us using crs 4326, which is the WGS 84 coordinate system. lat/lon conversion to x/y-coordinates alexschnale August 18, 2010, 2:15pm 1 mmr August 18, 2010, 5:50pm 2 alexschnale August 20, 2010, 8:43am 3. You need to re-project into a geographic system like WGS84 to convert to lat long. Please notice that the h is altitude above the WGS 84 ellipsoid. This sets the x and y to spatial coordinates. Type in the zone number or select from the list. View the results on this web page or fly there on Google Earth. Public Function GetSphericalLatitude(ByVal GPSLatitude As Double, ByVal h As Double) As Doubleĭim A As Double = 6378137 'semi-major axisĭim f As Double = 1 / 298.257223563 '1/f Reciprocal of flatteningĭim Rc As Double = A / (Sqrt(1 - e2 * (Sin(GPSLatitude * PI / 180) ^ 2)))ĭim p As Double = (Rc + h) * Cos(GPSLatitude * PI / 180)ĭim z As Double = (Rc * (1 - e2) + h) * Sin(GPSLatitude * PI / 180)ĭim SphericalLatitude As Double = Asin(z / r) * 180 / PI Convert Latitude and Longitude to State Plane Enter the Zone, Latitude, and Longitude. 'Input GPSLatitude is WGS84 Latitude,h is altitude above the WGS 84 ellipsoid

#Convert lat long to xy code

I attached a VB code I wrote: Imports System.Math

Latitude need be converted by WGS 84 ellipsoid parameters semi-major axis is 6378137 m, and.

Longitude in GPS（WGS84） and Cartesian coordinates are the same.

Theory for convert GPS（WGS84) to Cartesian coordinates Regarding, the "Haversine Formula" it is easy to implement and is nice because it is using "Spherical Trigonometry" instead of a "Law of Cosines" based approach which is based on two-dimensional trigonometry, therefore you get a nice balance of accuracy over complexity.Ī gentleman by the name of Chris Veness has a great website that explains some of the concepts you are interested in and demonstrates various programmatic implementations this should answer your x/y conversion question as well. Think "which point is closest?" scenarios where you don't need a precise distance measurement. For example, the result calculated from "Manhattan Distance Formula" versus the result from the "Distance Formula" can be better for certain situations as it is computationally less expensive. We need to convert from Lng/lat to XY and from XY to Lng/Lat.

#Convert lat long to xy software

I understand where starblue is coming from, but good software engineering is often about trade-offs, so it all depends on the accuracy you require for what you are doing. If you require a more rigidly WGS-84 compatible approach checkout the "Vincenty Formula." You will always have some amount of error unless you're talking about a distance of a few feet and even then there is theoretically curvature of the Earth. Yes, WGS-84 assumes the Earth is an ellipsoid, but I believe you only get about a 0.5% average error using an approach like the "Haversine Formula", which may be an acceptable amount of error in your case. "Haversine Formula" on WGS-84 data, which is a derivative of the "Law of Haversines" with very satisfying results. I have recently done something similar to this using the